Pythagoras and the early Pythagoreans thought that all numbers could be written as a fraction. While it’s trivially true that all (finite) decimal numbers can be expressed as a fraction – for example 1.23456789 is equal to 123456789/100000000 – and repeating fractions like 0.666… can also be expressed, there is another class of numbers which can’t be. These are the irrational numbers, so disliked by Mansfield, and include such important constants as pi and e.
The geometer Hippasus is often credited with the proof that the square root of two is one of these irrational numbers, but it’s more likely that he just leaked this information outside of the Pythagoreans. The Pythagoreans were indeed jealously secretive. Like the church killing Galileo in order to suppress the knowledge that the Earth moves around the Sun, the Pythagoreans are said to have drowned Hippasus to suppress the knowledge that some numbers could not be written as fractions. These seems a bit of an overreaction, especially from followers of a guy who invented the term for lover of knowledge. I guess philosophers can still be jealous lovers.
So what is the square root of 2? Using Pythagoras’ theorem, we can work it out. If we take a 1 by 1 square, the diagonal forms the hypotenuse of a right-angled triangle. Adding the squares on each of the other two sides gives us a total of 2 for the area of the square on the hypotenuse, and so its length is the square root of 2. If you measure it for yourself, you’ll find it’s approximately 1.4142 times the length of the side of the square.
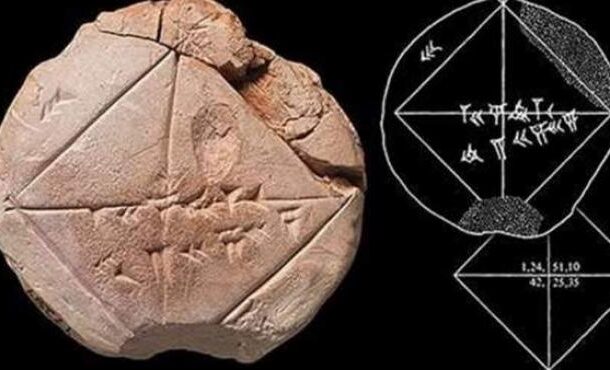
If you saw day 71, you won’t be surprised to find that Pythagoras wasn’t the first to calculate what is sometimes called Pythagoras’ number. It appears in the Shulba Sutras, Vedic texts written sometime in the 8th to the 6th centuries BC, but today’s tablet is still earlier. It was gifted to Yale’s Babylonian collection by J.P. Morgan, of unknown provenance, but based on the lentil shape and style of writing, most likely from the Old Babylonian (1800-1600 BC) period in southern Mesopotamia. The calculation, in the sexagesimal system, is accurate to 6 decimal places which is hugely impressive for the time.
I was surprised not to find more about this irrational number. It’s a pleasing value, and that kind of number tends to crop up all over the place. I only came across a couple of notable uses outside of complex geometry that I’m not about to try to summarise in a couple of sentences. From the ancient world, the ad quadratum method of designing courtyards developed by the Roman architect Vitruvius has the length of the space equal to the square root of 2 times the width. In more modern times, the ISO 216 standard for paper sizes (A4, A3, etc) uses the same aspect ratio of paper width to paper height.
And if you ever need to use the ratio yourself, you could approximate it to 1.4 like some sort of biblical pi (if you believe 1 Kings 7:23, pi is equal to 3), you could use the value from today’s tablet, which is close to 1.41421296, or even use 99/70 which is a close approximation as a fraction. But if accuracy is important, and you have the computing resources available to use it, NASA host the first million or so places so you can calculate away to your heart’s content. Just don’t let the Pythagoreans catch you doing it.

You stopped! Keep going, please – I just found this, and I’m on day 153 of my own challenge. I want to see you finish!